Building Understanding of Absolute Value
HS Algebra
Description
Explore the meaning of absolute value using visual representations, including dynagraphs and graphing on the coordinate plane.
Storyboard
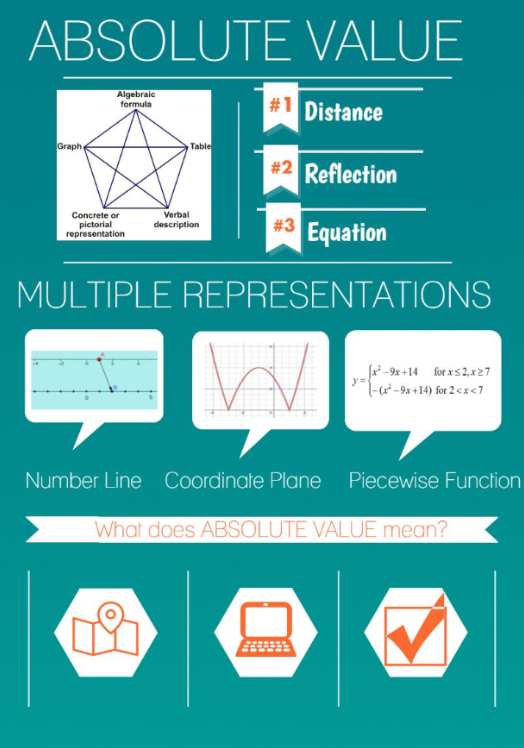
This ARC aims to create contexts for students to experience a variety of visual representations that reinforce the definition of absolute value. Explorations connecting back to elementary concepts and progressing in complexity provide intentional transitions for building deeper meaning. Representations considered in subsequent activities include the following topics:
- Number line - as the (positive) distance from zero.
- Double number lines - as functions in one-dimension.
- Coordinate Plane - as a graph of a piecewise function.
Audio
High-school teachers Tina, Janet, and Deidra reflect on their own learning and describe some 'aha moments' they experienced while working as a team to write this series of lessons.
Hook
How do you break a function into pieces?
Lessons
Lesson 1 of 3
Use dynagraphs to visualize and represent absolute value relationships.
Lesson 2 of 3
Students grapple with congruence through rigid transformations, then conjecture a "shortcut" set of conditions that also ensure congruence..
Lesson 3 of 3
Solving absolute value equations graphically and algebraically.
ARC Assessment
Technology Resources