By Harold Reiter, posted February 13, 2017 —

Failure to understand fractions and lack of fluency with their arithmetic often blocks students’ entry to algebra and higher-level mathematics. Perhaps wrestling with some interesting fraction problems can help. Here are three.

1. First, find a fraction between 1/2 and 2/3. Of course, many correct answers exist. I always ask my students to explain how they solved the problem. The most popular answer among teachers and middle school students is 7/12, the midpoint of the segment [1/2, 2/3], or the average of the original fractions. It is the most popular answer among people who have been taught how to solve problems like this. Among adults over 50 years of age, the most popular answer is 5/8, perhaps because they’ve worked with rulers graded in eighths, or possibly they were stock market investors before the big board went to penny increments for stocks.

My favorite two answers are 5/9 and 3/5. I got the answer 5/9 from a precocious six-year-old. He followed his answer with the explanation that 5/9 is greater than 5/10, which is 1/2, and 5/9 is less than 6/9, which is 2/3.

The wonderful answer 3/5 gives the teacher the opportunity to bring up the Water-Sharing model:

Ashley and Betty have a one-liter bottle of water to share; Carl, Dick, and Ethan have two one-liter bottles among the three of them. The girls each get less than each of the boys (1/2 < 2/3). But the five friends meet and agree to share all the water equally. How much does each person get now? 

The new fraction 3/5, called the mediant of 1/2 and 2/3, lies between 1/2 and 2/3. More generally, using the symbol  ⊕  to represent the mediant, we can write

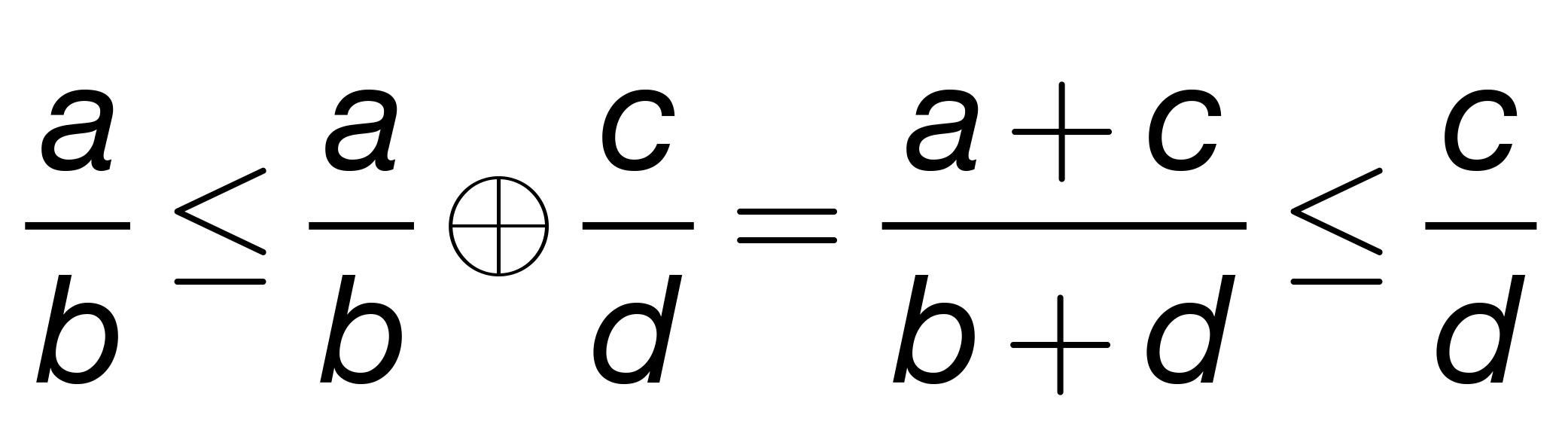
for positive integers a, b, c, and d with a/b < c/d. Note also that the mediant of two fractions depends on their representations and not their values. For example,

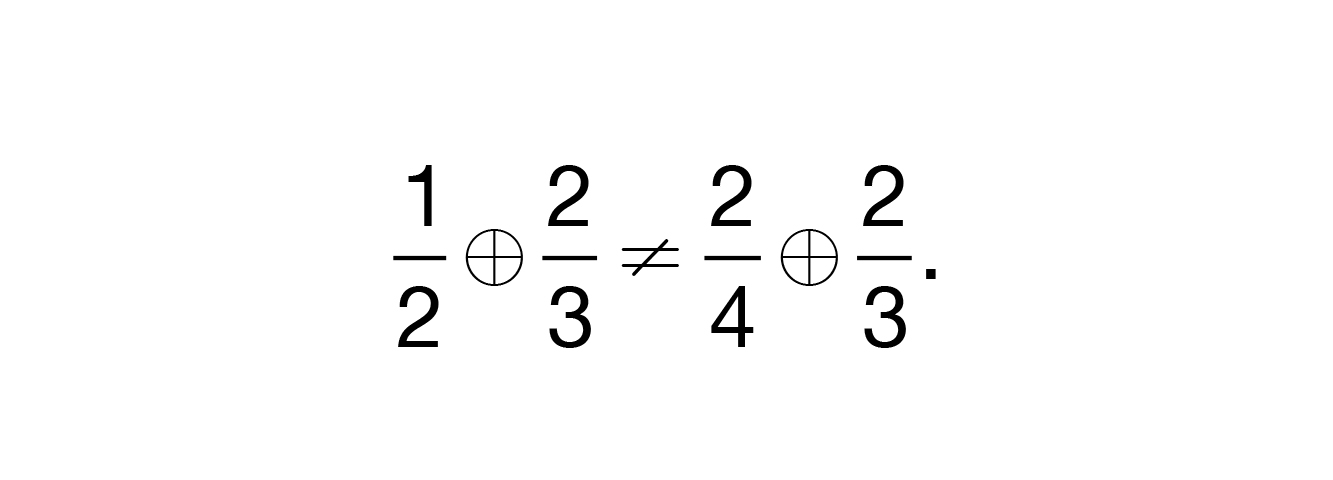
This oddity is the basis for the interesting Simpson’s paradox in probability and statistics. [Recent MT articles that address the mediant include “Mediants Make (Number) Sense of Fraction Foibles” by Eric L. McDowell, August 2016, p. 18; and “Curiosity and Connections” by Kien H. Lim, November 2014, p. 288.]

2. The second problem is actually a sequence of questions. First, ask your students to find a fraction a/b with the two properties 

•   a and b are different, one-digit numbers.

•   a/b is as large as possible but less than 1.

It won’t take long before students name 8/9 as the fraction. To prove the answer is best, recognize that 1 − a/b is as small as it can be.

Next, ask students to find four distinct a, b, c, and d so that a/b + c/d < 1 and the sum is otherwise as large as possible. Someone will realize that we cannot hope to do better than 71/72, so we should try solving a/8 + c/9 < 1, which succumbs to the Euclidean algorithm or trial and error. What about six different digits a, b, c, d, e, and f such that a/b + c/d + e/f is less than 1 but as large as possible? What about an eight-digit problem? (For eight digits, replace the upper bound 1 with a 2.)

3. Our final fraction problem comes from The Art of Problem Solving’s Beast Academy (https://www.beastacademy.com/), Book 5C. Find the next fraction in the sequence below:

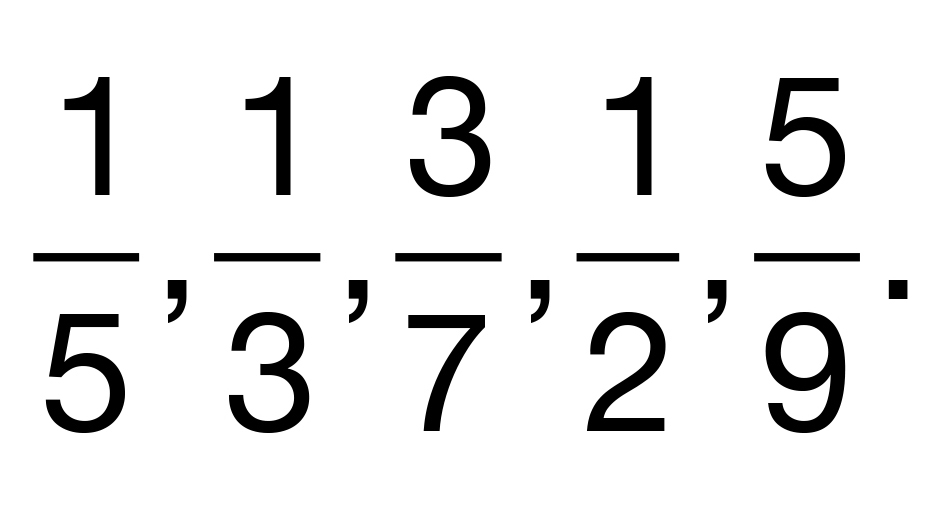
One of my fabulous fourth graders looked at the sequence of differences,

1/3 – 1/5 = 2/15

3/7 – 1/3 = 2/21

1/2 – 3/7 = 2/28

5/9 – 1/2 = 2/36

and recognized that that the denominators are all triangular numbers! The next difference should be 2/45, which makes the next in the sequence 2/45 + 5/9 = 3/5. With this additional value, we can perhaps see that the sequence of values is really just

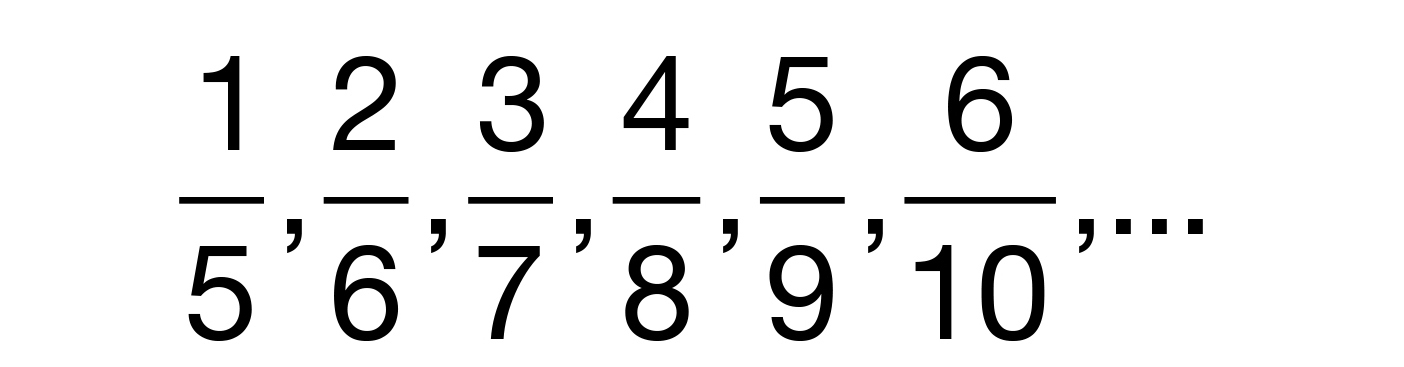
Harold Reiter has taught mathematics for more than fifty-two years. In recent years, he has enjoyed teaching at summer camps, including Epsilon, MathPath, and MathZoom. His favorite current activity is teaching fourth and fifth graders two days each week.