By Harold Reiter, posted January 30, 2017 —

With wooden cubes as manipulatives, I create problems related to pattern recognition, spatial visualization, polynomials, fractions, probability, and pretty combinations of these ideas. Today’s problems are about probability:

Build a 2 × 2 × 2 cube using eight unit cubes. Then imagine painting all six faces of the large cube. Cut the painted cube apart and randomly select one of the eight unit cubes, which you then roll like a die. What is the probability that a painted side comes up?

Most students will quickly reply with the answer of one-half. Then I can ask, “Where do we go from here?”

When I urge them to try the 1 × 1 × 1 cube 1 × 1 cube, they laugh and answer that the probability is 1. I ask, “What about the 3 × 3 × 3 cube?”

Some students realize that when the 3 × 3 × 3 cube is painted, four types of unit cubes are produced: 

•    1 with no painted sides

•    6 (we’ll call face cubes) with one side painted

•    12 edge cubes with two painted sides

•    8 corner cubes with three painted sides

This cube classification leads to the probability

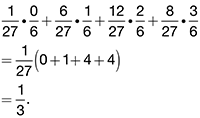
For the cubes with volumes 1, 8, and 27, we find the probabilities 1, 1/2, and 1/3. This might not be a coincidence. What’s next? Some students will dive right into the 4 × 4 × 4 case. Others will begin to ponder a method easier than cube classification. 

Every one of the 27 • 6 = 162 sides is equally likely to be selected, but only 54 sides are painted, because each of the six faces has area 9. Now (9 • 6)/(27 • 6) = 1/3, and we can see pretty quickly that in the general case of n × n × n cubes, we get (6n²)/(6n³) = 1/n as the probability that a randomly selected face is painted.

From here, we talk about rectangular boxes. Build an a × b × c block of cubes, with a < b < c. We can ask the same question, or we can turn it around. Suppose the block is painted on all six of its faces, and once again a unit cube is randomly selected: Find all triplets (a, b, c) that would yield the probability 2/7 that a painted face comes up. 

Some students will see right away that a = b = c is impossible because 2/7 is not a unit fraction. (What should I tell my little fourth-grade friend Lucio, who tells me that a 3.5 × 3.5 × 3.5 cube will work? He actually built such a cube from cardboard at home!)

Giving students time to experiment and think is important at this stage. Learning to tolerate confusion keeps them from saying, “But wait, you haven’t taught us how to do that kind of problem.” After students understand that all the sides are equally likely, they do not need to focus on the individual cubes. So, the number of sides of all the cubes is 6abc, and the number of painted sides is 2(ab + bc + ac). Setting this fraction equal to 2/7 and simplifying the equation results in

But a = 1 makes the left side too big, and a = 3 makes the left side too small (unless a = b = c = 3, which doesn’t work). With a = 2, we can subtract 1/2 from both sides to yield

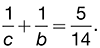
This has the unique integer solution {b = 3, c = 42}. 

(Ed note: For more investigation of the Painted Cube problem and probability, take a look at the Activities for Students department article “What’s in a Cube” in the upcoming March 2017 issue of MT.)

Harold Reiter has taught mathematics for more than fifty-two years. In recent years, he has enjoyed teaching at summer camps, including Epsilon, MathPath, and MathZoom. His favorite current activity is teaching fourth and fifth graders two days each week.