Looking Squarely at the Difference!  
Here’s a “kick back and enjoy” summertime Problem to Ponder: Which whole numbers can be expressed as the difference of two perfect squares? 

Some Ponderings: 

The July Problem to Ponder posed the following question: Which whole numbers can be expressed as the difference of two perfect squares?

Students sometimes begin problems like these by making a list. The list might start out rather haphazardly, but often when we organize a list, we can make some useful observations. For example, suppose that we made a list like the following:

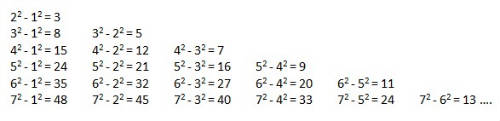
We might notice that the top diagonal consists of all the odd numbers. We note also that 1 is the difference of the whole numbers 1² – 0², and the next diagonal includes all the multiples of 4, and we can also get 4 itself from 2² - 0². Also, we notice that some whole numbers can be expressed as the difference of two perfect square whole numbers in more than one way, like 5² - 1² = 24 = 7² - 5², and 4² - 1² = 15 = 8² - 7².

One way to show that we can generate all the odd numbers as the difference of perfect squares is that the difference of two consecutive perfect square whole numbers is 
(n + 1) ² – (n) ²  =  n² + 2n + 1 - n²   =  2n + 1, so as n ranges over {0, 1, 2, 3, 4, …}, every odd number can be expressed as the difference of two perfect square whole numbers.

We can also represent the odd numbers, as well as other numbers, as the differences of perfect squares visually on grids. In each case below, a shaded perfect square grid is cut out from the corner of a larger perfect square grid, leaving a shape that represents the difference of two perfect square whole numbers.

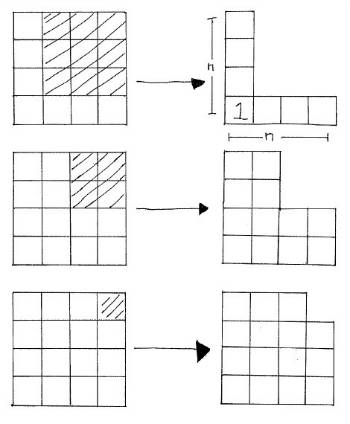
Differences of consecutive squares make L shapes that we can see as “2n + 1.” Removing smaller perfect squares leads to “fatter” L shapes. Our problem then becomes, Which whole numbers can be represented as “L-shaped numbers”? We can clearly represent all the odd numbers as L shapes on a grid. The question then is, Can we also represent all the even numbers as L shapes too?

More ponderings on this problem next month…

Further Ponderings:

The July Problem to Ponder posed the following question: Which whole numbers can be expressed as the difference of two perfect squares?

In our previous discussion, we noted that every odd number could be expressed as the difference of two consecutive perfect squares—that is, as (n + 1) ² – (n) ²  = 2n + 1, as n ranges over the set of whole numbers. We also noted that the difference of perfect squares can be represented visually, with a smaller square cut away from a corner of the larger square, leaving an L-shaped number, as shown in the figure below.

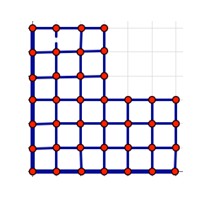
The question left for us was, Which even numbers can be represented as L-shaped numbers? We can partition any of these L shapes into three regions—a corner square and two congruent rectangles, as follows:

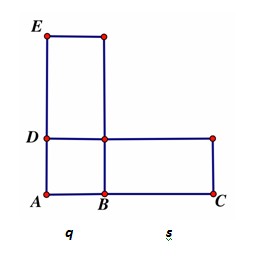
Suppose that the length of AB = q, and the length of BC = s.

If L is the area in square units of this L-shape, then L = q² + 2qs = q(q + 2s).

 If L is to be an even number, then it must be the case that q is also even. For if q were odd, then q + 2s would be odd (odd + even = odd), and so q (q + 2s) would be odd, which would mean that L was odd—but we started with an even number L—a contradiction.

Since q must also be even when L is even, q = 2r for some whole number r, and so this means that

L = q(q + 2s) = (2r)(2r + 2s) = 4(r)(r + s).

Thus, only even numbers that are multiples of 4 can be expressed as the difference of two perfect square whole numbers. Now the question is, Given any number that is a multiple of 4, could we in fact write it as the difference of two perfect squares?

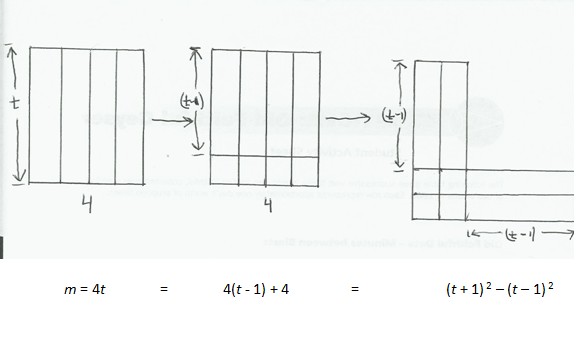
This series of diagrams shows how any number m that is multiple of 4 (that is, m = 4t for some whole number t) can be expressed as the difference of two perfect squares. Any multiple of 4 can be made into an L shape that is the difference of two perfect squares.