By Victoria Bill and Laurie Speranzo, posted July 17, 2017 —

When students talk about their solution paths and others’ solution paths, they have opportunities to make sense of ideas and, therefore, have some ownership of them as well. The students in the classroom vignette below are learning a great deal, because they are talking about models and making sense of mathematics. The teacher is also learning about student thinking and reasoning. See what you understand about these students' understanding for division of a fraction by a fraction.

Students are explaining that 1/2 divided by 1/4 equals 2. Their discussion follows:

Jessica: It is two, because if you think about a half of a cake, you want to figure out how many fourths are in the half-cake, and there are two of them.

Ms. Clark: Who understood and can put the idea into their own words?

Jason:  She said there is half, and inside half—if you cut it into two pieces—there are two smaller pieces.

Aisha: Those two pieces are fourths.

Ms. Clark: Can anyone add on to what she said?

Sean: Can I make a drawing? You can do one-fourth times four, and you get two of them.

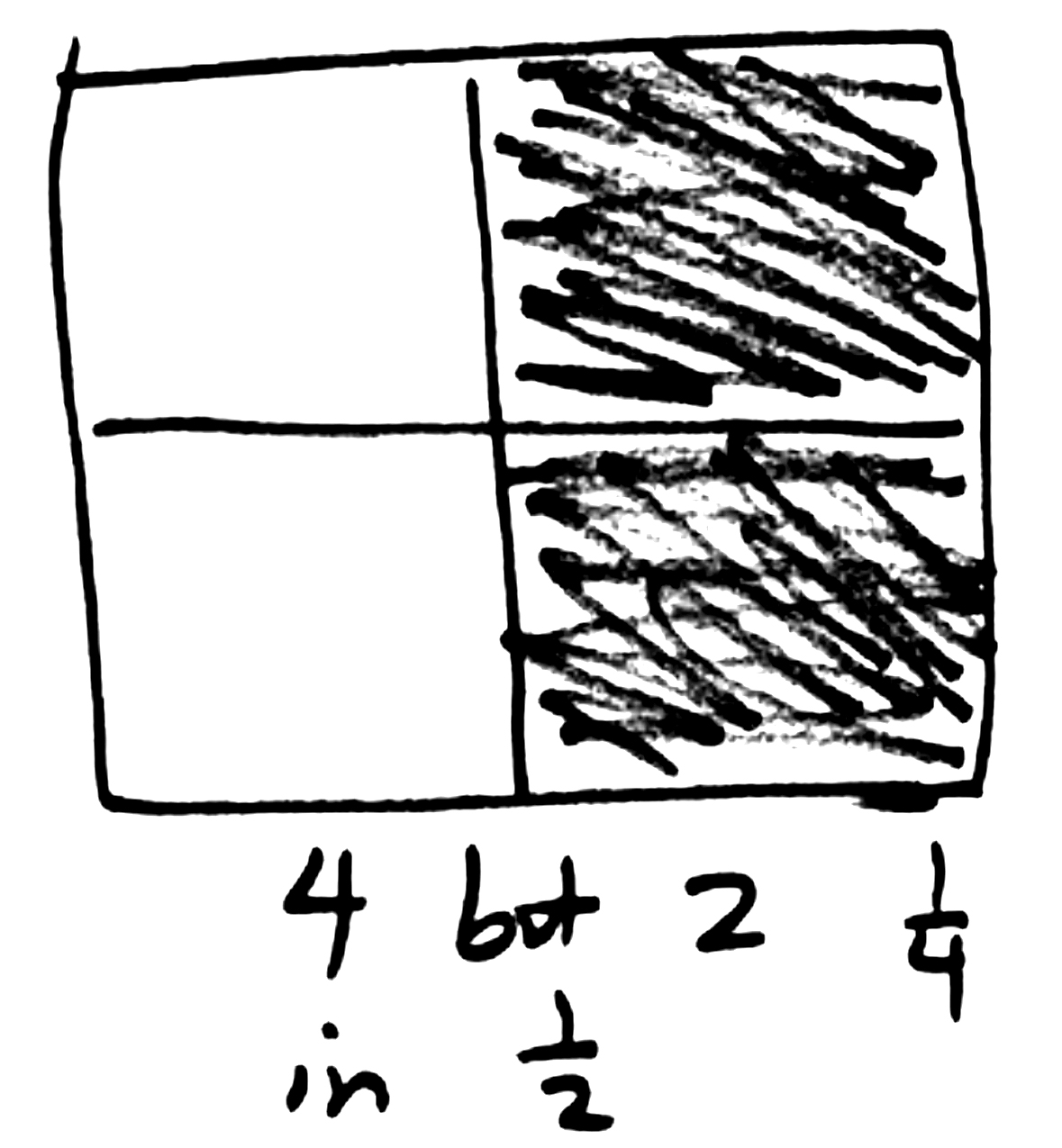
Ms. Clark: I think I understand your drawing. Who can come up, point to Sean’s drawing, and explain one more time what he said?

Juan: I don’t understand why you did that.

Ms. Clark: Who can explain what he did?

Jason: He knows that fourths mean there will be four pieces, so he is figuring out how many fourths are in the whole and the half.

Sean: There are four pieces in the whole, but we only have half, so this means now there are two-fourths in the half.

Ms. Clark: I need to hear that back a few more times to make sure I understand the thinking.

We know it sounds trivial, but more and more, we are convinced that when a student shares his or her work, all students benefit from follow-up opportunities for between five and eight students to—

• say back what they heard,
• add on,
• make a drawing,
• say it in their own words,
• agree or disagree, and
• summarize the ideas.  

Had the teacher moved forward and just accepted the first student’s response, many other students in the classroom might not have understood or have been totally clear about why the quotient to 1/2 ÷ 1/4 = 2. The teacher would also have missed an opportunity to learn more about how her students were thinking about the problem. 

The Institute for Learning* calls the process of hearing from many students in the classroom, talk that is “accountable to community.” Imagine the teacher has a student who created the following number line to solve 1/2 ÷ 1/4:

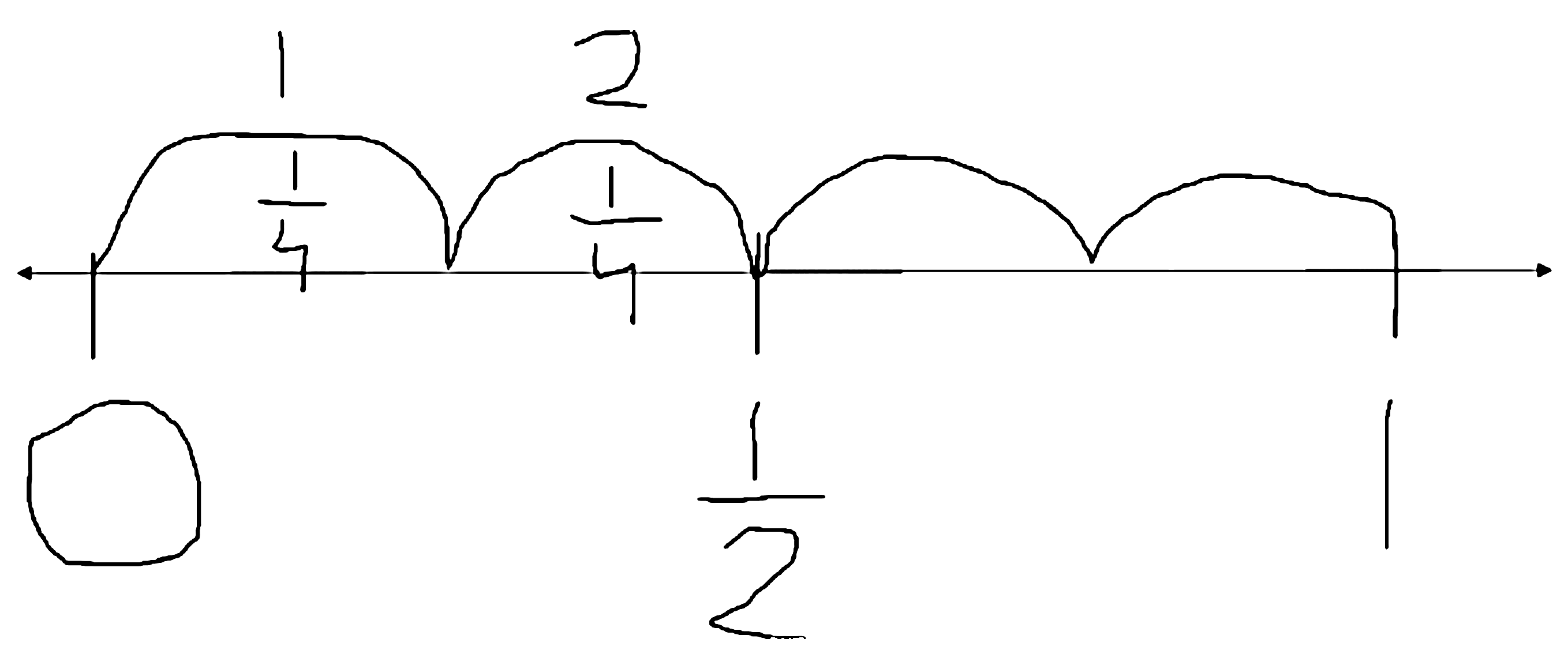
This visual model looks incredibly different from the area model first introduced. A different representation of the whole is shown with two one-fourths contained within the one-half. We suggest that at least five to eight students would need to point to and explain what is happening with the number line model before the teacher can be sure that all the students have a true understanding of the math in the model.

Only after discussing both models can the teacher finally ask students to compare and contrast the models. If you do not understand each solution path, you cannot make comparisons between solution paths. By asking students to say more, to add on, to agree or disagree with their peer’s solution paths, the teacher can focus on the uptake of student ideas (Applebee et al. 2003; Nystrand 2006; Soter et al. 2008).

Engle and Conant (2002) show that productive disciplinary engagement occurs in learning environments characterized by (1) giving students authority to address problems, (2) holding students accountable to others and to shared disciplinary norms, (3) problematizing subject matter by asking students to compare and contrast solution paths, and (4) providing students with relevant resources, such as context, manipulatives, or the freedom to make diagrams or number lines.

Your Turn

Engage students in solving and discussing the solution paths to a high-level mathematics task. If the task is a high-level task, students will have multiple ways to solve the task. Remember, when you have a solution path shared, you should call on five to eight students by using the questions above. This means you may use a question more than once. Write and tell all of us what happens in your classroom as several students say and say again in their own words what they heard. Please share in the comments section or reach out to Victoria Bill or Laurie Speranzo.

References

Applebee, Arthur N., Judith A. Langer, Martin Nystrand, and Adam Gamoran. 2003. “Discussion-Based Approaches to Developing Understanding: Classroom Instruction and Student Performance in Middle and High School English.” American Educational Research Journal 40, no. 3 (Autumn): 685–730.

Nystrand, Martin. 2006). “Research on the Role of Classroom Discourse as It Affects Reading Comprehension.” Research in the Teaching of English 40, no. 4 (May): 392–412.

Soter, Anna O., Ian A. Wilkinson, P. Karen Murphy, Lucila Rudge, Kristin Reninger, and Margaret Edwards, M. 2008. “What the Discourse Tells Us: Talk and Indicators of High-Level Comprehension.” International Journal of Educational Research 47 (6): 372–91.

Engle, Randi A., Faith R. Conant. 2002. “Guiding Principles for Fostering Productive Disciplinary Engagement: Explaining an Emergent Argument in a Community of Learners Classroom.” Cognition and Instruction 20 (4): 399–483.

The Institute for Learning (IFL) is an outreach of the University of Pittsburgh's Learning Research and Development Center (LRDC). Comprising scholar practitioners, the IFL helps educators bring what research tells us about teaching and learning into classrooms to help students grow their intelligence and reach the high standards demanded by today’s colleges and workforce. We believe—and research confirms—that virtually all students are capable of high achievement, if they work hard at the right kinds of learning tasks.

Victoria Bill is a Resident Fellow with IFL at the Learning Research and Development Center, University of Pittsburgh.  She also co-authored with DeAnn Huinker the 2017 NCTM publication Taking Action: Implementing the Effective Teaching Practices in Grades Pre-K–5. Laurie Speranzo is a resident fellow with IFL at the Learning Research and Development Center, University of Pittsburgh. In addition to her work at IFL, she has recently been appointed to serve on the editorial panel of NCTM’s Mathematics Teaching in the Middle School journal.